出典: フリー百科事典『ウィキペディア(Wikipedia)』
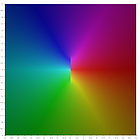
6つの逆双曲線関数 (arsinh , arcosh , artanh , arcoth , arsech , arcsch ) のグラフ 逆双曲線関数 (ぎゃくそうきょくせんかんすう、英語 : inverse hyperbolic functions )は、数学 において与えられた双曲線関数 の値に対応して双曲角 (英語版 ) 関数 。双曲角の大きさは双曲線 x y = 1に対応する双曲的扇形 (英語版 ) 面積 に等しく、単位円 の扇形 の面積は対応する中心角 の2分の1 である。一部の研究者は逆双曲線関数のことを、双曲角を明確に理解するため「面積関数」(英語 : area function )と呼ぶ。
逆双曲線関数を表す略記法 arsinh やarcosh とは異なる略記法として、arcsinh やarccosh などが本来誤表記であるにもかかわらず良く使用されるのだが、接頭辞 arc はarcus (弓 )の省略形であり、接頭辞ar はarea の省略形である[ 1] [ 2] [ 3] 計算機科学 の分野では、しばしばasinh という省略形を用いる。累乗 を表す上付き文字−1と誤解しないように注意を払う必要があるという事実にもかかわらず、sinh−1 (x ), cosh−1 (x ), などの略記も用いられる。また、cosh−1 (x ) とcosh(x )−1 は似て非なるものである。
各関数は複素数平面 で次のように定義される。
arsinh
z
=
ln
(
z
+
z
2
+
1
)
arcosh
z
=
ln
(
z
+
z
+
1
z
−
1
)
artanh
z
=
1
2
ln
(
1
+
z
1
−
z
)
arcoth
z
=
1
2
ln
(
z
+
1
z
−
1
)
arcsch
z
=
ln
(
1
z
+
1
z
2
+
1
)
arsech
z
=
ln
(
1
z
+
1
z
+
1
1
z
−
1
)
{\displaystyle {\begin{aligned}\operatorname {arsinh} \,z&=\ln(z+{\sqrt {z^{2}+1}}\,)\\[2.5ex]\operatorname {arcosh} \,z&=\ln(z+{\sqrt {z+1}}{\sqrt {z-1}}\,)\\[1.5ex]\operatorname {artanh} \,z&={\tfrac {1}{2}}\ln \left({\frac {1+z}{1-z}}\right)\\\operatorname {arcoth} \,z&={\tfrac {1}{2}}\ln \left({\frac {z+1}{z-1}}\right)\\\operatorname {arcsch} \,z&=\ln \left({\frac {1}{z}}+{\sqrt {{\frac {1}{z^{2}}}+1}}\,\right)\\\operatorname {arsech} \,z&=\ln \left({\frac {1}{z}}+{\sqrt {{\frac {1}{z}}+1}}\,{\sqrt {{\frac {1}{z}}-1}}\,\right)\end{aligned}}}
上記の平方根 は正の平方根であり、対数関数 は複素対数 である。実数の引数 、例えばz = xは実数値を返すが、一定の簡素化を行うことが可能であり、例えば
x
+
1
x
−
1
=
x
2
−
1
{\displaystyle {\sqrt {x+1}}{\sqrt {x-1}}={\sqrt {x^{2}-1}}}
上記の関数は次のように級数展開できる。
arsinh
x
=
x
−
(
1
2
)
x
3
3
+
(
1
⋅
3
2
⋅
4
)
x
5
5
−
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
x
7
7
+
⋯
=
∑
n
=
0
∞
(
(
−
1
)
n
(
2
n
)
!
2
2
n
(
n
!
)
2
)
x
2
n
+
1
(
2
n
+
1
)
,
|
x
|
<
1
{\displaystyle {\begin{aligned}\operatorname {arsinh} \,x&=x-\left({\frac {1}{2}}\right){\frac {x^{3}}{3}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{5}}{5}}-\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{7}}{7}}+\cdots \\&=\sum _{n=0}^{\infty }\left({\frac {(-1)^{n}(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{2n+1}}{(2n+1)}},\qquad \left|x\right|<1\end{aligned}}}
arcosh
x
=
ln
2
x
−
(
(
1
2
)
x
−
2
2
+
(
1
⋅
3
2
⋅
4
)
x
−
4
4
+
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
x
−
6
6
+
⋯
)
=
ln
2
x
−
∑
n
=
1
∞
(
(
2
n
)
!
2
2
n
(
n
!
)
2
)
x
−
2
n
(
2
n
)
,
x
>
1
{\displaystyle {\begin{aligned}\operatorname {arcosh} \,x&=\ln 2x-\left(\left({\frac {1}{2}}\right){\frac {x^{-2}}{2}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{-4}}{4}}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{-6}}{6}}+\cdots \right)\\&=\ln 2x-\sum _{n=1}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{-2n}}{(2n)}},\qquad x>1\end{aligned}}}
artanh
x
=
x
+
x
3
3
+
x
5
5
+
x
7
7
+
⋯
=
∑
n
=
0
∞
x
2
n
+
1
(
2
n
+
1
)
,
|
x
|
<
1
{\displaystyle {\begin{aligned}\operatorname {artanh} \,x&=x+{\frac {x^{3}}{3}}+{\frac {x^{5}}{5}}+{\frac {x^{7}}{7}}+\cdots \\&=\sum _{n=0}^{\infty }{\frac {x^{2n+1}}{(2n+1)}},\qquad \left|x\right|<1\end{aligned}}}
arcsch
x
=
arsinh
1
x
=
x
−
1
−
(
1
2
)
x
−
3
3
+
(
1
⋅
3
2
⋅
4
)
x
−
5
5
−
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
x
−
7
7
+
⋯
=
∑
n
=
0
∞
(
(
−
1
)
n
(
2
n
)
!
2
2
n
(
n
!
)
2
)
x
−
(
2
n
+
1
)
(
2
n
+
1
)
,
|
x
|
>
1
{\displaystyle {\begin{aligned}\operatorname {arcsch} \,x=\operatorname {arsinh} {\frac {1}{x}}&=x^{-1}-\left({\frac {1}{2}}\right){\frac {x^{-3}}{3}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{-5}}{5}}-\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{-7}}{7}}+\cdots \\&=\sum _{n=0}^{\infty }\left({\frac {(-1)^{n}(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{-(2n+1)}}{(2n+1)}},\qquad \left|x\right|>1\end{aligned}}}
arsech
x
=
arcosh
1
x
=
ln
2
x
−
(
(
1
2
)
x
2
2
+
(
1
⋅
3
2
⋅
4
)
x
4
4
+
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
x
6
6
+
⋯
)
=
ln
2
x
−
∑
n
=
1
∞
(
(
2
n
)
!
2
2
n
(
n
!
)
2
)
x
2
n
2
n
,
0
<
x
≤
1
{\displaystyle {\begin{aligned}\operatorname {arsech} \,x=\operatorname {arcosh} {\frac {1}{x}}&=\ln {\frac {2}{x}}-\left(\left({\frac {1}{2}}\right){\frac {x^{2}}{2}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{4}}{4}}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{6}}{6}}+\cdots \right)\\&=\ln {\frac {2}{x}}-\sum _{n=1}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{2n}}{2n}},\qquad 0<x\leq 1\end{aligned}}}
arcoth
x
=
artanh
1
x
=
x
−
1
+
x
−
3
3
+
x
−
5
5
+
x
−
7
7
+
⋯
=
∑
n
=
0
∞
x
−
(
2
n
+
1
)
(
2
n
+
1
)
,
|
x
|
>
1
{\displaystyle {\begin{aligned}\operatorname {arcoth} \,x=\operatorname {artanh} {\frac {1}{x}}&=x^{-1}+{\frac {x^{-3}}{3}}+{\frac {x^{-5}}{5}}+{\frac {x^{-7}}{7}}+\cdots \\&=\sum _{n=0}^{\infty }{\frac {x^{-(2n+1)}}{(2n+1)}},\qquad \left|x\right|>1\end{aligned}}}
またオイラー によるarctanの展開[ 4]
artanh
x
=
∑
n
=
0
∞
2
2
n
(
n
!
)
2
(
2
n
+
1
)
!
(
−
1
)
n
x
2
n
+
1
(
1
−
x
2
)
n
+
1
,
|
x
|
<
1
2
{\displaystyle \operatorname {artanh} \,x=\sum _{n=0}^{\infty }{\frac {2^{2n}(n!)^{2}}{(2n+1)!}}{\frac {(-1)^{n}x^{2n+1}}{(1-x^{2})^{n+1}}},\qquad \left|x\right|<{\frac {1}{\sqrt {2}}}}
(
arsinh
x
)
2
=
∑
n
=
0
∞
2
2
n
+
1
(
n
!
)
2
(
2
n
+
2
)
!
(
−
1
)
n
x
2
n
+
2
,
|
x
|
<
1
{\displaystyle (\operatorname {arsinh} \,x)^{2}=\sum _{n=0}^{\infty }{\frac {2^{2n+1}(n!)^{2}}{(2n+2)!}}(-1)^{n}x^{2n+2},\qquad \left|x\right|<1}
arsinh x に対する漸近展開 は次の式で与えられる。
arsinh
x
=
ln
2
x
+
∑
n
=
1
∞
(
−
1
)
n
−
1
(
2
n
−
1
)
!
!
2
n
(
2
n
)
!
!
1
x
2
n
{\displaystyle \operatorname {arsinh} \,x=\ln 2x+\sum \limits _{n=1}^{\infty }{\left({-1}\right)^{n-1}{\frac {\left({2n-1}\right)!!}{2n\left({2n}\right)!!}}}{\frac {1}{x^{2n}}}}
d
d
x
arsinh
x
=
1
1
+
x
2
d
d
x
arcosh
x
=
1
x
2
−
1
d
d
x
artanh
x
=
1
1
−
x
2
d
d
x
arcoth
x
=
1
1
−
x
2
d
d
x
arsech
x
=
−
1
x
(
x
+
1
)
1
−
x
1
+
x
d
d
x
arcsch
x
=
−
1
x
2
1
+
1
x
2
{\displaystyle {\begin{aligned}{\frac {d}{dx}}\operatorname {arsinh} \,x&{}={\frac {1}{\sqrt {1+x^{2}}}}\\{\frac {d}{dx}}\operatorname {arcosh} \,x&{}={\frac {1}{\sqrt {x^{2}-1}}}\\{\frac {d}{dx}}\operatorname {artanh} \,x&{}={\frac {1}{1-x^{2}}}\\{\frac {d}{dx}}\operatorname {arcoth} \,x&{}={\frac {1}{1-x^{2}}}\\{\frac {d}{dx}}\operatorname {arsech} \,x&{}={\frac {-1}{x(x+1)\,{\sqrt {\frac {1-x}{1+x}}}}}\\{\frac {d}{dx}}\operatorname {arcsch} \,x&{}={\frac {-1}{x^{2}\,{\sqrt {1+{\frac {1}{x^{2}}}}}}}\\\end{aligned}}}
実数x に対して、
d
d
x
arsech
x
=
∓
1
x
1
−
x
2
;
ℜ
{
x
}
≷
0
d
d
x
arcsch
x
=
∓
1
x
1
+
x
2
;
ℜ
{
x
}
≷
0
{\displaystyle {\begin{aligned}{\frac {d}{dx}}\operatorname {arsech} \,x&{}={\frac {\mp 1}{x\,{\sqrt {1-x^{2}}}}};\qquad \Re \{x\}\gtrless 0\\{\frac {d}{dx}}\operatorname {arcsch} \,x&{}={\frac {\mp 1}{x\,{\sqrt {1+x^{2}}}}};\qquad \Re \{x\}\gtrless 0\end{aligned}}}
微分法 の例:θ = arsinh x とおくと、
d
arsinh
x
d
x
=
d
θ
d
sinh
θ
=
1
cosh
θ
=
1
1
+
sinh
2
θ
=
1
1
+
x
2
{\displaystyle {\frac {d\,\operatorname {arsinh} \,x}{dx}}={\frac {d\theta }{d\sinh \theta }}={\frac {1}{\cosh \theta }}={\frac {1}{\sqrt {1+\sinh ^{2}\theta }}}={\frac {1}{\sqrt {1+x^{2}}}}}
sinh
(
arcosh
x
)
=
x
2
−
1
for
|
x
|
>
1
sinh
(
artanh
x
)
=
x
1
−
x
2
for
|
x
|
<
1
cosh
(
arsinh
x
)
=
1
+
x
2
cosh
(
artanh
x
)
=
1
1
−
x
2
for
|
x
|
<
1
tanh
(
arsinh
x
)
=
x
1
+
x
2
tanh
(
arcosh
x
)
=
x
2
−
1
x
for
|
x
|
>
1
{\displaystyle {\begin{aligned}\sinh(\operatorname {arcosh} x)&={\sqrt {x^{2}-1}}&{\text{for }}|x|>1\\\sinh(\operatorname {artanh} x)&={\frac {x}{\sqrt {1-x^{2}}}}&{\text{for }}|x|<1\\\cosh(\operatorname {arsinh} x)&={\sqrt {1+x^{2}}}\\\cosh(\operatorname {artanh} x)&={\frac {1}{\sqrt {1-x^{2}}}}&{\text{for }}|x|<1\\\tanh(\operatorname {arsinh} x)&={\frac {x}{\sqrt {1+x^{2}}}}\\\tanh(\operatorname {arcosh} x)&={\frac {\sqrt {x^{2}-1}}{x}}&{\text{for }}|x|>1\end{aligned}}}
arsinh
u
±
arsinh
v
=
arsinh
(
u
1
+
v
2
±
v
1
+
u
2
)
arcosh
u
±
arcosh
v
=
arcosh
(
u
v
±
(
u
2
−
1
)
(
v
2
−
1
)
)
artanh
u
±
artanh
v
=
artanh
(
u
±
v
1
±
u
v
)
arsinh
u
+
arcosh
v
=
arsinh
(
u
v
+
(
1
+
u
2
)
(
v
2
−
1
)
)
=
arcosh
(
v
1
+
u
2
+
u
v
2
−
1
)
{\displaystyle {\begin{aligned}\operatorname {arsinh} u\pm \operatorname {arsinh} v&=\operatorname {arsinh} \left(u{\sqrt {1+v^{2}}}\pm v{\sqrt {1+u^{2}}}\right)\\\operatorname {arcosh} u\pm \operatorname {arcosh} v&=\operatorname {arcosh} \left(uv\pm {\sqrt {\left(u^{2}-1\right)\left(v^{2}-1\right)}}\right)\\\operatorname {artanh} u\pm \operatorname {artanh} v&=\operatorname {artanh} \left({\frac {u\pm v}{1\pm uv}}\right)\\\operatorname {arsinh} u+\operatorname {arcosh} v&=\operatorname {arsinh} \left(uv+{\sqrt {\left(1+u^{2}\right)\left(v^{2}-1\right)}}\right)\\&=\operatorname {arcosh} \left(v{\sqrt {1+u^{2}}}+u{\sqrt {v^{2}-1}}\right)\end{aligned}}}
arcosh
(
2
x
2
−
1
)
=
2
arcosh
(
x
)
for
x
≥
1
arcosh
(
8
x
4
−
8
x
2
+
1
)
=
4
arcosh
(
x
)
for
x
≥
1
arcosh
(
2
x
2
+
1
)
=
2
arsinh
(
x
)
for
x
≥
0
arcosh
(
8
x
4
+
8
x
2
+
1
)
=
4
arsinh
(
x
)
for
x
≥
0
{\displaystyle {\begin{aligned}\operatorname {arcosh} (2x^{2}-1)&=2\operatorname {arcosh} (x)&{\text{ for }}x\geq 1\\\operatorname {arcosh} (8x^{4}-8x^{2}+1)&=4\operatorname {arcosh} (x)&{\text{ for }}x\geq 1\\\operatorname {arcosh} (2x^{2}+1)&=2\operatorname {arsinh} (x)&{\text{ for }}x\geq 0\\\operatorname {arcosh} (8x^{4}+8x^{2}+1)&=4\operatorname {arsinh} (x)&{\text{ for }}x\geq 0\end{aligned}}}
^ Jan Gullberg , Mathematics: From the Birth of Numbers (New York : W. W. Norton & Company , 1997 ), ISBN 0-393-04002-X , p. 539には以下のような記述がある。
arcsinh x , arccosh x などの似て非なる表記法は、厳しく糾弾されなければならない。実際これらの関数はarc とは何らの関係もなく、ar eaと関係するものであり、それはラテン語で書かれた真の名前が証明している。
arsinh = ラテン語 : area sinus hyperbolicus ラテン語 : area cosinus hyperbolicus
^ Eberhard Zeidler, Wolfgang Hackbusch and Hans Rudolf Schwarz, Oxford Users' Guide to Mathematics (Bruce Hunt英訳, Oxford : Oxford University Press , 2004 ), ISBN 0-19-850763-1 , Section 0.2.13: "The inverse hyperbolic functions", p. 68には以下のような記述がある。
「
逆双曲線関数のラテン語名は、area sinus hyperbolicus, area cosinus hyperbolicus, area tangens hyperbolicusそしてarea cotangens hyperbolicus (x ). ...である。
」
上記の引用では、arsinh, arcosh, artanh, arcothをそれぞれの逆双曲線関数の表記法として採用している。
^ Ilja N. Bronshtein, Konstantin A. Semendyayev, Gerhard Musiol and Heiner Muehlig, Handbook of Mathematics (Berlin : Springer-Verlag , 5th ed., 2007 ), ISBN 3-540-72121-5 , doi :10.1007/978-3-540-72122-2 , Section 2.10: "Area Functions", p. 91には以下のような記述がある。
面積関数 は双曲線関数の逆関数すなわち逆双曲線関数 である。関数sinh x , tanh x およびcoth x は狭義単調関数であるので、何らの制限事項も設けることなく各々が逆関数を持つ。関数cosh x の定義域は2つの単調な(無限)区間に分けられるので、二つの逆関数を考えることができる。また名前にある 面積 と言う語は、この関数の幾何学的な定義が特定の双曲的扇形の面積であるという事実を意味している。...
^ Chien-Lih, Hwang (2005). “89.67 An Elementary Derivation of Euler's Series for the Arctangent Function” . The Mathematical Gazette 89 (516): 469–470. ISSN 0025-5572 . https://www.jstor.org/stable/3621947 .
Weisstein, Eric W. "Inverse hyperbolic functions" . mathworld.wolfram.com (英語). area functions PlanetMath .(英語) Hazewinkel, Michiel, ed. (2001), “Inverse hyperbolic functions” , Encyclopedia of Mathematics ISBN 978-1-55608-010-4 , https://www.encyclopediaofmath.org/index.php?title=Inverse_hyperbolic_functions Hazewinkel, Michiel, ed. (2001), “Area-function” , Encyclopedia of Mathematics ISBN 978-1-55608-010-4 , https://www.encyclopediaofmath.org/index.php?title=Area-function Inverse hyperbolic functions [リンク切れ - University College London Department of Mathematics2.22 逆双曲線関数 [リンク切れ - 同志社大学 ・近藤弘一

![{\displaystyle {\begin{aligned}\operatorname {arsinh} \,z&=\ln(z+{\sqrt {z^{2}+1}}\,)\\[2.5ex]\operatorname {arcosh} \,z&=\ln(z+{\sqrt {z+1}}{\sqrt {z-1}}\,)\\[1.5ex]\operatorname {artanh} \,z&={\tfrac {1}{2}}\ln \left({\frac {1+z}{1-z}}\right)\\\operatorname {arcoth} \,z&={\tfrac {1}{2}}\ln \left({\frac {z+1}{z-1}}\right)\\\operatorname {arcsch} \,z&=\ln \left({\frac {1}{z}}+{\sqrt {{\frac {1}{z^{2}}}+1}}\,\right)\\\operatorname {arsech} \,z&=\ln \left({\frac {1}{z}}+{\sqrt {{\frac {1}{z}}+1}}\,{\sqrt {{\frac {1}{z}}-1}}\,\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/95f2128eda727211b5638f59d57b696a2dbff434)